Coppock Curve for DJIA Getting Up to High Level
Free Chart In Focus email
Delivered to you every week
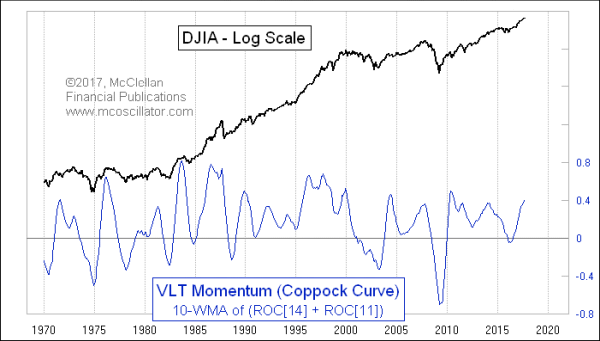
Years ago, a technical analyst and money manager named E.S.C. (Sedge) Coppock created an indicator that has since come to be known as the “Coppock Curve”. Coppock never called it that himself, instead calling it his Very Long Term (VLT) Momentum Index. That indicator is now getting up to a high level, which says that the uptrend is getting stretched.
What Coppock wanted was to have an indicator that would help identify the really juicy bottoming conditions which only appear every few years. It shows that when it goes to a deeply negative value and then turns up, and that upturn is key. When it is below zero and falling, that is typically a pretty bad time for stock prices.
Technicians also use it for other purposes, as an identifier of the trend direction generally. In this respect, it can be somewhat problematic, since prices sometimes continue rising for a while after the Coppock Curve turns down. And like any indication of trend direction, it can be subject to whipsaws.
Coppock chose the rather unique math for his indicator in an interesting way. He was managing money for an Episcopal church, and in a conversation with one of the priests Coppock learned that the typical grief period after the loss of a loved one takes a person around 11-14 months. Coppock figured that the same sort of feelings of grief were involved in how people felt about losses they suffered with their investments. So he combined the 11-month and 14-month percentage changes in the DJIA, and then ran a 10-month weighted moving average (WMA) of that data to get the indicator you see above.
In a 10-month WMA, the most recent month’s data gets a weighting of 10, then the prior month 9, then 8, 7, etc. going back to 10 months back which gets a weighting of just 1. You then divide by the sum of those weighting factors, which is 55. An indicator developer nowadays might employ different math to get at the heart of that 11-14 month grieving period, because a WMA is somewhat more difficult to calculate (although my computer does not seem to care). But we should understand that Coppock developed this idea back in the 1960s, when the use of moving averages of any type was still a new idea, especially for the financial markets.
With a little bit more math, we can calculate the price at which the Coppock Curve would turn down. I call that the “Coppock Unchanged” number, because if the DJIA were to close at exactly that value, then the Coppock Curve would be exactly unchanged. A close below it means that the Coppock Curve turns down. I’ll spare you the full derivation of that formula, as it is a little bit intensive, but it is shown in column H of this spreadsheet. See also Calculating The Coppock Curve.
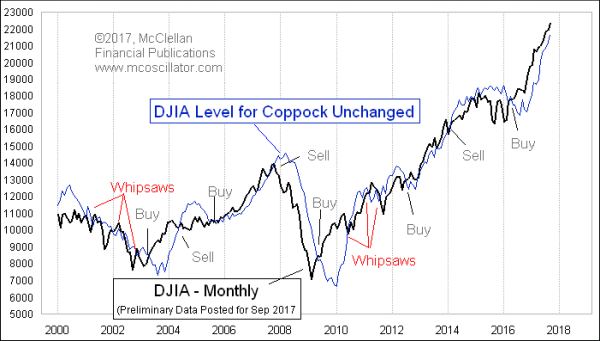
The Coppock Unchanged line is racing up faster than the DJIA, and so at some point we are going to see prices cross below it. As noted in the chart, this can be a good sell signal sometimes, and it can also be subject to whipsaws. The Coppock Unchanged value for September is 21,678.94, so the DJIA would have to drop pretty hard on Sep. 29, the final trading day of the month in order to trigger this signal. But for October, that number goes up to around 22,400 (depending on September’s monthly close), so a signal gets easier to see with each passing month.
I noted above that these Coppock Curve downturn sell signals can sometimes be misleading. What can be said is that when the Coppock Curve gets up to a high level, the uptrend is getting pretty stretched, and the gains get harder to come by. That is something we already know from the stretched valuations, but it is nice to see it in another way too.
Tom McClellan
Editor, The McClellan Market Report
Feb 06, 2014
Coppock Curve Turns Down |
Sep 04, 2015
DJIA’s Proportional Price Oscillator |
Jun 23, 2017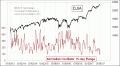
Narrow Range for McClellan Oscillator |